SUPPLEMENTARY PROBLEMS

1. A 50 l b block of ice is on a ramp inclined at 20⁰. What force pushing up the ramp is required to keep the block from sliding? What is the actual force caused by the weight of ice against the ramp?

   Ans. 171 l b and 47 l b

2. A man can row his boat at 3 mph. He is in a steam flowing from north to south at 4 mph. How fast and in what direction will he travel if rows i) directly downstream ii) directly upstream iii) due east (across the stream) iv) North East (45⁰ on a navigator campass)

   Ans i) 7mph.s ii)1 mph.s iii) 5 mph; 143.130⁰ iv) 2.8336 mph; 131.529⁰

3. A petrol boat leaves its base on a heading of 17⁰ travelling at 17 km/hr. After 2 hours it turns to new heading of 310⁰ and slows to 12 km/hr after 45 minutes on this new heading. How far and in what direction is the boat from its base.

   Ans. 38.420 km, 4.5476⁰

4. A manual lawnmover requires a force of 10 lbs parallel to the ground in order to move it through the grass; if the handle is inclined at 41⁰ to the ground. How much force is necessary along the handle?

   Ans. 13.2501 l bs

5. In a plane add 120 N Force at 30⁰ and a -100 N force at 90⁰ using the parallelogram method.

   Ans. R= 11/N , q = 339⁰ with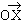

   

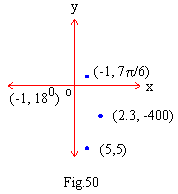
6. Plot the points with the given polar coordinates

   (i) (-1, 18⁰ ) (ii) (2.3, -400) (iii) (-1, 7p /6 rad) (iv) (5, 5rad)

   Ans.

7. Find the set of polar coordinates for the points with given Cartesian coordinates. (i) (-3, 0)    (ii) (2, 2Ö3)    (iii) (5, -12)    (iv) ( 1, -3)

   Ans. i) (3, 180⁰)     (ii) (4, 60⁰)   (iii) (13, -67.38⁰   (iv) ( Ö10, -71.57⁰)

8. Convert the equations to polar form   (i) x = 7   (ii) x²+ y² = 9   (iii) y = 2x   (iv) x²- y² = 1   (v)  

   Ans.   (i) r cos q =7   (ii) r = 3   (iii) q = tan-1 (2)   (iv) r² cos 2q = 1   (v) r² (cos² q + 4 sin² q ) = 4

9. Convert  the  equations  to  Cartesian coordinates       (i) r = 3   (ii) q = 3p/4   (iii) r sin q =7   (iv) r² cos q = 3 sin q - cos q

   Ans.    (i)   x² + y² = 9     (ii)   y = -x     (iii)   y = 7 (iv)   (x² + y² ) x = 3y- x

   *********

   [next page]

Index

7.1 Scalers & Vectors
7.2 Algebra of Vectors
7.3 Representation of a vector in a plane
7.4 Dotor Scalar product
7.5 Polar Co-ordinates
Supplementary Problems

Chapter 8