|
7.3 Representation of a Vector in a plane
Let P (x, y) be any point on the coordinate plane. 
Any such vector can be broken down into two components, a 


Here x and y are components of p along x-axis and y-axis respectively. This ordered pair (x , y) associated with p is known as the rectangular components of p.
Let ÐPOX = q then
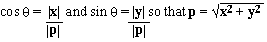
which is the magnitude of p.
This concept can be extended to higher dimensions such as three dimension.
Also, note that any vector 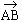 can be shifted so that its initial point A is set at 'O', then can be shifted so that its initial point A is set at 'O', then 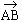 is in 'standard position'. Now is in 'standard position'. Now 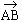 = =  then then  replaces replaces 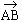 . .
Thus  is the standard vector in the plane for all vectors in the plane of the same magnitude and direction as is the standard vector in the plane for all vectors in the plane of the same magnitude and direction as  . .
\ OP = OM + ON

If coordinates of A are (xa, ya) and that of B are (xb, yb) then shifting A to O and making B = P we have coordinates of P are (xb - xa, yb - ya)
\ 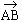 or or  º (xb - xa,
yb - ya) º (xb - xa,
yb - ya)
Thus an algebraic vector is an ordered pair of real numbers that corresponds to a standard geometric vector  . .
|