Example 53

The radius of a sphere is measured as 24 cm with an error of 0.01cm. Find (I) the approximate error (II) relative error and (III) percentage error in calculating its volume.

Solution : If r is the radius of the sphere, then its volume is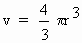

\

\ approximate error in volume (dv) =

\ when r = 24 and dr = 0.01

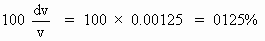
Example 54

The time T of a complete oscillation of a simple pendulum of length l is given by , where g is a constant. If there is an error of 2% in the value of l , find the

approximate error and percentage error in the calculated value of T.

[next chapter]

Index

5.1 Tangent And Normal Lines
5.2 Angle Between Two Curves
5.3 Interpretation Of The Sign Of The Derivative
5.4 Locality Increasing Or Decreasing Functions 5.5 Critical Points
5.6 Turning Points
5.7 Extreme Value Theorem
5.8 The Mean-value Theorem
5.9 First Derivative Test For Local Extrema
5.10 Second Derivative Test For Local Extrema
5.11 Stationary Points
5.12 Concavity And Points Of Inflection 5.13 Rate Measure (distance, Velocity And Acceleration)
5.14 Related Rates
5.15 Differentials : Errors And Approximation

Chapter 6