Composite function : Let f : A ® B and g : B® C be two functions and x be any element of A. Then f(x) Î B. Let y = f(x) Since B is the domain of the function g and C is its co-domain, g(y) Î C. Let z = g (y) then z = g (y) = g[f(x)]Î C. This shows that every element x of set A related to unique element z = g [f(x)] of C. This gives rise to a function from the set A to the set C. This function is called the composite of f and g. It is denoted by ‘g of ’ and we have

If f : A® B and g : B® C are two functions then the composite of f and g is the function gof : A® C given by

(gof) (x) = g [ f(x) ] x Î A

A function ' f ' is such that f (x + p) = f(x) x or f(x) and f(x + p) are both undefined, then p is the period of f :

For example, ' sin e ' has period 2p rad. Since sin (x+2p) = sin x x

Linear function: f(x) = ax + b, a ¹ 0 is called a linear function. The highest degree of x is 1. It is always one-one onto.

Now we know that a linear equation in y variables can be expressed in the form:

ax + by + c = 0, a ¹ 0 , b ¹ 0. This represents a straight line. An important part of a straight line is its slope 'm'. It is a number of units the line climbs (or falls) vertically for each unit of horizontal change from left to right.

Thus slope (m)

=

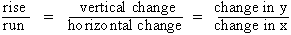
\ m = but Dy = y₁ - y₂ and Dx = x₁-x2

\ m = or

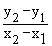
Note that :

1. slope of a horizontal line or any line parallel to x-axis is always 0, as vertical change remains 0 and horizontal change remains constant.

2. slope of a vertical line or any line parallel to y-axis, is said to be undefined, in other words, it has no slope. Since horizontal change (i.e. y) remains zero with vertical change (i.e. x) value remains constant.

3. If q indicates the inclination of an oblique line with then slope (m) = tan q.

4. If q is acute, the line has slope (m) which is positive and if q is obtuse, line has slope (m) which is negative.

5. If two straight lines are parallel we have their slopes being equal, i.e. [ m₁ = m₂ ] and two straight lines are mutually perpendicular,the product of their slopes equals to -1, i.e. [ m₁ · m₂ = -1 ] Þ m₁ =