\
A (x) = area of the equilateral triangle
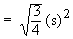
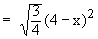
\ The volume of the solid is given by


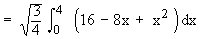
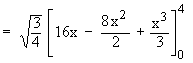
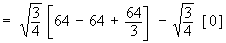
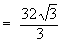
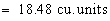
(II) Volumes of Solids of Revolutions
With the help of the definite integral, one can find the volume of a solid, it can be done by revolving a plane region about a horizontal or a vertical line, which does not pass through the plane region.
Such solids are made up of one of the three types of elements viz. disk, washers or cylindrical shells. Each type has its own approach, that is different from the others, in setting up the definite integral to determine its volume.
[next page]
|