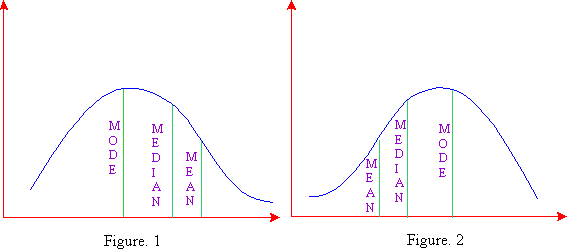
A distribution in which the values of mean, median and mode coincide (i.e. mean = median = mode) is known as a symmetrical distribution. Conversely, when values of mean, median and mode are not equal the distribution is known as asymmetrical or skewed distribution. In moderately skewed or asymmetrical distribution a very important relationship exists among these three measures of central tendency. In such distributions the distance between the mean and median is about one-third of the distance between the mean and mode, as will be clear from the diagrams 1 and 2. Karl Pearson expressed this relationship as:

Mode = mean - 3 [mean - median]

Mode = 3 median - 2 mean

and Median = mode +

Knowing any two values, the third can be computed.

Example
Given median = 20.6, mode = 26 Find mean.

Solution:

   

Index

4.1 Introduction
4.2 Arithmetic Mean
4.3 Properties of Arithmetic Mean
4.4 Median
4.5 Mode
4.6 Empirical relation between mean, median & mode

Chapter 5